A lot of students prepping for Quant, especially those students away from math for a long time, get lost when trying to divide by a square root. However, dividing by square roots is not something that should intimidate you. With a short refresher course, you’ll be able to divide by square roots in no time. First, consider these three practice questions.
Q1.A.
B.
C.
D.
E.

A.
B.
C.
D.
E.
A.
B.
C.
D.
E.
The second one throws in a little geometry. You may want to review the properties of the
When we first met fractions, in our tender prepubescence, both the numerators and denominators were nice easy positive integers. As we now understand, any kind of real number, any number on the entire number line, can appear in the numerator or denominator of a fraction. Among other things, radicals —- that is, square-root expressions — can appear in either the numerator or denominator. There’s no particular issue if we have the square-root in a numerator. For example,
is a perfectly good fraction. In fact, those of you who ever took trigonometry might even recognize this special fraction. Suppose, though, we have a square root in the denominator: what then? Let’s take the reciprocal of this fraction.
This is no longer a perfectly good fraction. Mathematically, this is a fraction “in poor taste”, because we are dividing by a square-root. This fraction is crying out for some kind of simplification. How do we simplify this?
By standard mathematical convention, a convention the GMAT follows, we don’t leave square-roots in the denominator of a fraction. If a square-root appears in the denominator of a fraction, we follow a procedure called rationalizing the denominator.
We know that any square root times itself equals a positive integer. Thus, if we multiplied a denominator of the square root of 3 by itself, it would be 3, no longer a radical. The trouble is — we can’t go around multiplying the denominator of fractions by something, leaving the numerator alone, and expect the fraction to maintain its value. BUT, remember the time-honored fraction trick — we can always multiply a fraction by
Thus, to simplify a fraction with the square root of 3 in the denominator, we multiply by the square root of 3 over the square root of 3!
That last expression is numerically equal to the first expression, but unlike the first, it is now in mathematical “good taste”, because there’s no square root in the denominator. The denominator has been rationalized (that is to say, the fraction is now a rational number).
Sometimes, some canceling occurs between the number in the original numerator and the whole number that results from rationalizing the denominator. Consider the following example:
That pattern of canceling in the simplification process may give you some insight into practice problem #1 above.
This is the next level of complexity when it comes to dividing by square roots. Suppose we are dividing a number by an expression that involves adding or subtracting a square root. For example, consider this fraction:
This is a fraction in need of rationalization. BUT, if we just multiply the denominator by itself, that WILL NOT eliminate the square root — rather, it will simply create a more complicated expression involving a square root. Instead, we use the difference of two squares formula,
Notice that the multiplication in the denominator resulted in a “differences of two squares” simplification that cleared the square roots from the denominator. That final term is a fully rationalized and fully simplified version of the original.
Having read these posts about dividing by square roots, you may want to give the three practice questions at the top of this article another try, before reading the explanations below. If you have any questions on dividing by square roots or the explanations below, please ask them in the comments sections! And good luck conquering these during your QUANT!
because, in general, we can multiply and divide through "Simplifying Radical Expressions".
Cross-multiplying, we get
You may well have found this and wondered why it’s not listed as an answer. This is numerically equal to the correct answer, but of course, as this post explains, this form is not rationalized. We need to rationalize the denominator.
Answer = D
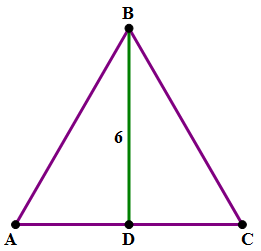
Q2. We know the height of ABC and we need to find the base. Well, altitude BD divides triangle ABC into two 30-60-90 triangles. From the proportions in a 30-60-90 triangle, we know:
Now, my predilection would be to rationalize the denominator right away.
Now,
Answer = C
Q3. We start by dividing by the expression in parentheses to isolate
Of course, this form does not appear among the answer choices. Again, we need to rationalize the denominator, and this case is a little trickier because we have addition in the denominator along with the square root. Here we need to find the conjugate of the denominator — changing the plus sign to a minus sign — and then multiply the numerator and denominator by this conjugate. This will result in —
Answer = A